Chapter 4.
The Row
Brindle’s Categorisations
Most 12-tone composition books emphasise a few points to consider when composing a tone row. For the sake of completion and comprehensive understanding I’ll summarise very briefly what Brindle has written in Serial Composition (p. 4-17) regarding distinguishing different types of tone rows.
Melodic Series
The first two types of rows that he describes he titles ‘melodic series,’ and ‘tonal series.’ He uses the former distinction to indicate a row that has an ‘expressive melodic arc.’ I think a fair comparison of the ‘melodic series,’ can be made to George Pearle’s ‘pitch-cell’ compositions. These row’s intervallic choices function almost like leitmotifs throughout the composition.
Berg’s Lyric Suite
![]()
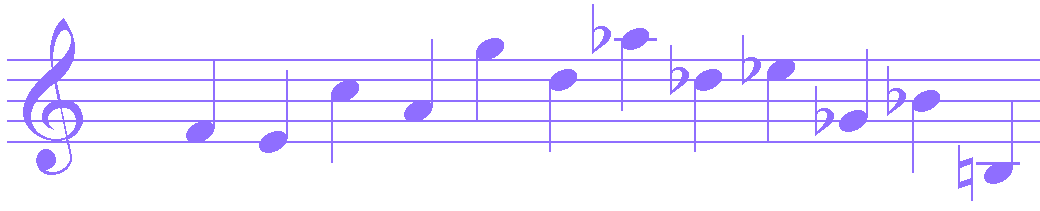
Brindle’s distinction between the first two types of series is indeed miniscule. He writes that in much of the music of Berg and Dallapiccola ‘...it is obvious that on many occasions the melodic character of the series has been a prime consideration.’ Later he goes on to describe the above tone-row indicating that Berg’s intention was to use a series ‘...with strong lyrical possibilities.’ How this differs from the following ‘tonal series’ is not made clear. He does however go on to highlight how certain rows (ex. Dallapiccola’s Il Prigioniero ‘Hope series’ and ‘Liberty series’) were written to express and represent certain emotions and are used by the composer to do just that.
Tonal Series
The second type of row Bridle describes is the ‘tonal series.’ These rows exemplify elements of tonality, perhaps pitch sequences that outline or imply certain chords, or utilise tonicising cadential figures. Rows that utilise whole-tone and octatonic patterns are also included in this section.
Secondary tone-row from Benjamin Frankel’s Curse of the Werewolf
![]()
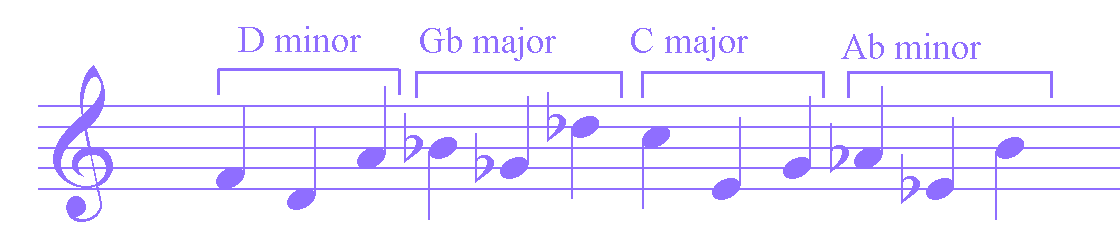
Frankel uses this row to build tension before an attack. The sequential triads retain the serialised nature of the music while their juxtaposition of unrelated keys works well to build suspense in various scenes.
One frequently cited piece that uses a strong ‘tonal series,’ is Berg’s Violin Concerto (1935).
Berg’s Violin Concerto
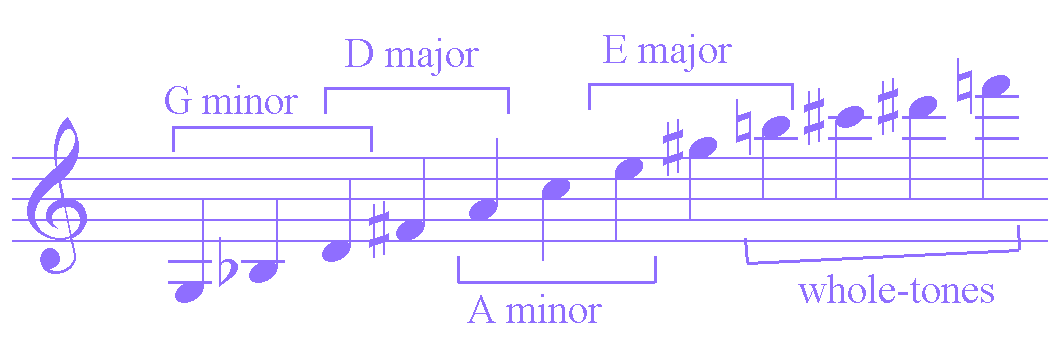
He advertently constructed the tone row out of major and minor triads, which could easily lead to melodic monotony. Intervallically, he only has these intervals to work with:
M2, m3, M3
To avoid this potential for monotony he treats the row with considerable freedom.
You’ll also notice that unlike the Frankel set which utilises triads that are rather distantly related, Berg constructed his set using triads that are very closely related. Throughout the piece he is careful to avoid feelings of dominant to tonic relationships that could be easily exposed if the row were played in retrograde.
Atonal Series
The next row category is the ‘Atonal Series.’ Without going into too much detail here, the ‘atonal series’ is constructed in such a way as to avoid any tonal implications. For example, no triads or chord implications, groups of wholetone relationships, consecutive 4ths/5ths, and no successive note groupings that could imply a chromatic chord (ex. diminished seventh chord).
Permissible three note cluster in the ‘atonal series.’





Naturally these three note clusters could (with the exception of #1 and #3) fit into a key. The main point is that they are not triadic in nature and don’t imply any singular key. This lack of implication is important to their function within a row.
Webern was quite fond of this type of row and used it in many compositions to great effect. One such example is from his Variations for Piano Op. 27.
Variations for Piano Op. 27.

Variations for Piano not only exploits this ‘atonal series’ but also shows great examples of symmetry within the piece. In this particular example, Webern effectively eliminates any of the tonal directions in music and introduces us to a truly unique and new sounding vocabulary.
It needs to be mentioned that using an ‘atonal series’ doesn’t necessarily beget an atonal sounding result. Similarly, using a ‘tonal series’ doesn’t necessarily produce a tonal sounding result.
Below is the row used in Lou Harrison’s Symphony in G:
Symphony in G
![]()

Take notice that if we look at the notes in groups of threes, few traditionally harmonic triads are readily apparent. There is an outlined A major chord in pitches 9, 10, 11, and we can extend this to include pitch 8, a D, which extends this harmony to be a D major 7 chord. With these as the exception the other three note groupings that could imply a key, when combined with a fourth note from the row (from either side of the group) are immediately disavowed of this notion. To be sure, this isn’t 100% an ‘atonal series,’ but one worth mentioning and listening to because it illustrates a very clear difference compared with the examples of the ‘tonal series’ above.
Harrison achieves rather tonal sounds with his permutations of this tone-row by using many different forms of the row as well as by starting on pitches in the middle of the row so as to suit his desired harmonic impetus. In relatively short compositions he would often use upwards of 10 row forms, a strategy that certainly bears further examination.
I consider the ‘atonal series’ to be far more delicate than the ‘tonal series.’ If we stray too far from the atonal series or treat it with too much freedom then its innate characteristics are lost, it ceases to be an atonal series. Whereas the tonal series can be treated with considerable freedom, and can essentially still maintain its core traits.
A very generalised statement could be made as such:
The more a row resembles a ‘tonal series,’ the freer we need to treat it. The more a row resembles an ‘atonal series,’ the less free we should treat it.
The more a row resembles a ‘tonal series,’ the freer we need to treat it. The more a row resembles an ‘atonal series,’ the less free we should treat it.
Symmetrical Series
This brings us to the ‘symmetrical series.’ Brindle uses this term to indicate rows that have portions which are symmetrical in their interval structure. The simplest example would be to show one point of symmetry in the middle of the row.
Ex. D E Eb C# F C | F# B G A Bb Ab